http://en.wikipedia.org/wiki/Aleph_number
start there and adjust your theory some more.
There exists a set of numbers that all divide down to one.
There exists a set of numbers that all divide into the prime.
Therefore there are two sets that numbers divide into 1 and prime.
Points can be organized into lines to planes to volumes to times.
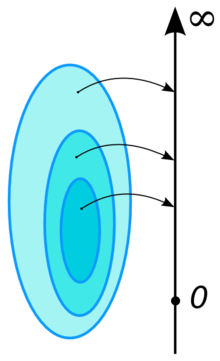
A sphere is bubble like and at present is divided into 360x360x360 to denote volume.
In this sense only are all spheres regardless of size of the same size.
That fact only defines the notion of scale invariability.
The size of the point is increased/decreased in linear, planar and volumetics only.
It says nothing about the measure it merely denotes the units of that system.
Thank Symptom for helping us to find this syntactical device.
P.S. The same also applies to the inverses of each number in the cardinal series?

No comments:
Post a Comment